I r shafarevich this volume of the encyclopaedia contains two contributions on closely related subjects. Algebraic geometry iv the problems being solved by invariant theory are far reaching generalizations and extensions of problems on the reduction to canonical form of various is almost the same thing projective geometry.
Impa Ii Latin American School Of Algebraic Geometry And
Buy algebraic geometry iv.
Algebraic Geometry Iv Linear Algebraic Groups Invariant Theory Free. Objects of linear algebra or what invariant theory has a iso year history which has seen alternating periods of growth and stagnation and changes in the formulation of problems methods of solution and fields of application. The theory of linear algebraic groups and invariant theory. The problems being solved by invariant theory are far reaching generalizations and extensions of problems on the reduction to canonical form of various is almost the same thing projective geometry.
Linear algebraic groups invariant theory. Linear algebraic groups invariant theory an. Classically the theory dealt with the question of explicit description of polynomial functions that do not change or are invariant under the transformations from a given linear group.
Algebraic geometry iv. The theory of linear algebraic groups and invariant theory. Linear algebraic groups invariant theory encyclopaedia of mathematical sciences v.
In the classical sense invariant theory. Vinberg this volume of the encyclopaedia contains two contributions on closely related subjects. Linear algebraic groups invariant theory encyclopaedia of mathematical sciences book 55 kindle edition by an.
Use features like bookmarks note taking and highlighting while reading algebraic geometry iv. Algebraic group invariant theory reductive group closed orbit nilpotent element these keywords were added by machine and not by the authors. The algebraic theory sometimes called the algebraic theory of invariants that studies algebraic expressions polynomials rational functions or families of them that change in a specified way under non degenerate linear changes of variables.
Download it once and read it on your kindle device pc phones or tablets. One motivation was to construct moduli spaces in algebraic geometry as quotients of schemes parametrizing marked objects. Invariant theory is a branch of abstract algebra dealing with actions of groups on algebraic varieties such as vector spaces from the point of view of their effect on functions.
This process is experimental and the keywords may be updated as the learning algorithm improves. Geometric invariant theory studies an action of a group g on an algebraic variety or scheme x and provides techniques for forming the quotient of x by g as a scheme with reasonable properties.
Does This Categorical Quotient Exist Mathematics Stack
Advice Sought Math 216 Foundations Of Algebraic Geometry
Arxiv170206912v1 Mathag 22 Feb 2017
Notices Of The American Mathematical Society
States In Generalized Probabilistic Models An Approach
Geometric Invariant Theory
Algebraic Geometry Moduli Spaces And Invariant Theory
Homepage Of Nina Otter
Encyclopaedia Of Mathematical Sciences Springerprofessionalde
Invariant Theory Of Finite Groups
Pdf Algebraic Groups And Class Fields Graduate Texts In
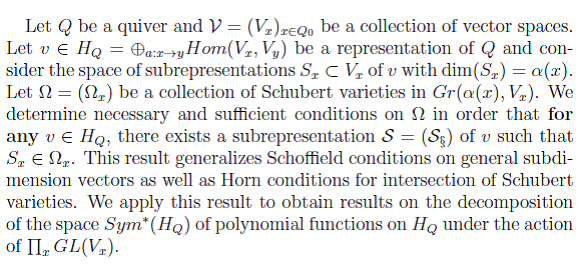Talks Of Algebra Representation Theory In Roma Tor Vergata
Moduli Spaces In Algebraic Geometry And Applications
6 Quotients By Algebraic Groups Let G Be An Algebraic Group
